Automating Traditional Methods of Shape Analysis….
Krumbien / Sloss Chart
What is the Krumbien / Sloss Chart?
William Christian Krumbein (1902–1979) was an established Geologist who was known to pursue innovative methods in Geology using extensive mathematics. An award was named after him, the Krumbein Medal of the International Association for Mathematical Geology (IAMG). This award was established at the 25th International Geological Congress in Sydney, in 1976.
Krumbien is also well known for his “Krumbein Scale” which was a means to measure the roundness, or sphericity, of particles. In 1963 he and L. Sloss published this scale in Stratigraphy and sedimentation. From then on, this was became better known as the Krumbien and Sloss Chart.
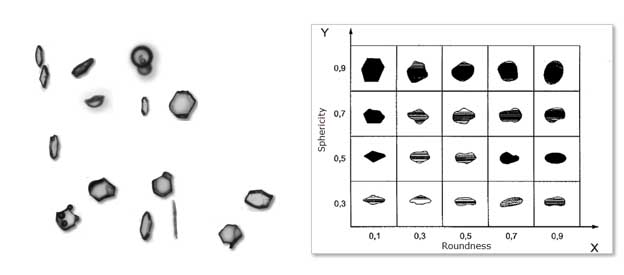
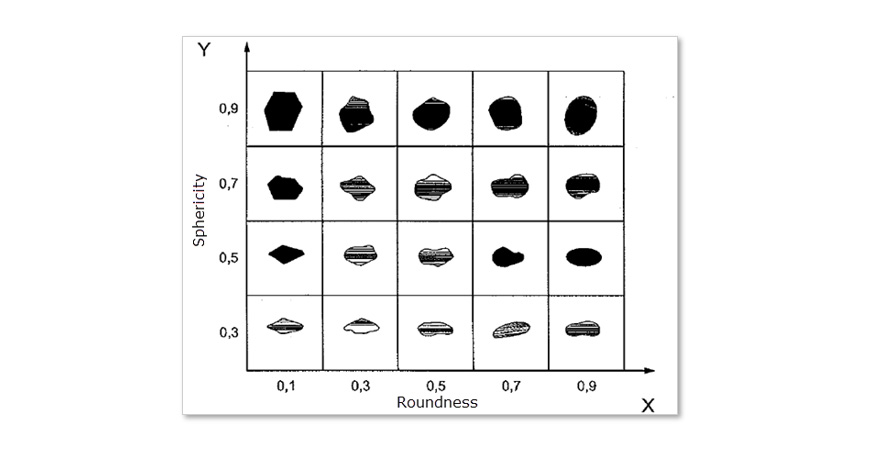
The chart has two axis. One is Sphericity on the Y axis and Roundness on the X axis. The chart has a series of particle images with different sizes and shapes. From researching several articles, it seems that the Sphericity on this chart is quantified as the diameter ratio between the largest inscribed and the smallest circumscribing sphere. Roundness is quantified as the average radius of curvature of surface features relative to the radius of the maximum sphere that can be inscribed in the
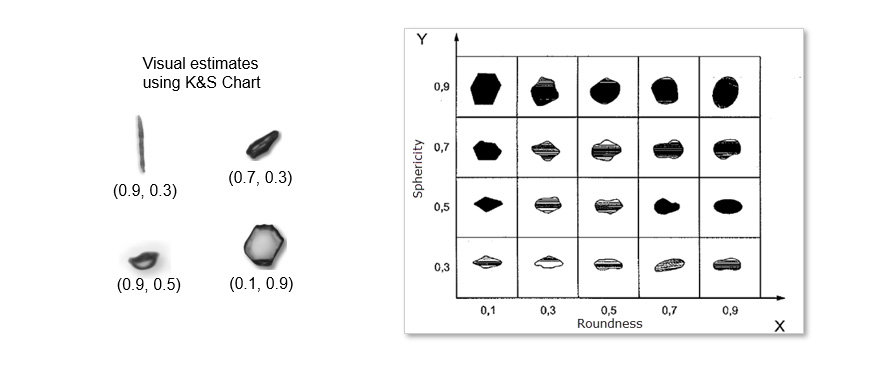
particle. In practical use of this chart, the Roundness and Sphericity of particles being analyzed are visually compared to the chart and are assigned a value based on this visual estimate.
W. C. Krumbein and L. L. Sloss, Stratigraphy and sedimentation, San Francisco, W. H. Freeman, 1963
Potential difficulties from visual comparisons
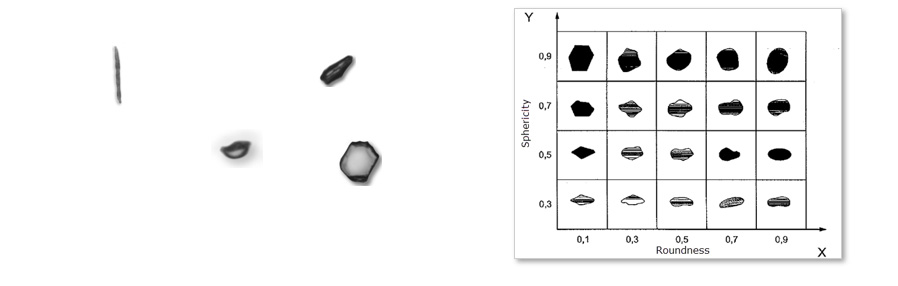
Back in 1963, the Krumbien and Sloss Chart was a breakthrough. It became a way to somewhat Quantify soils and sediments based on shape. When referring to a specific sample, a scientist could qualify a sample based on an estimated Sphericity and Roundness value when looking under a microscope. This was somewhat revolutionary. However, the method did have some possible issues. There was the user bias that this method would have. One person comparing particles to the K&S chart may be different than a different operator. Based on the need to use microscopy, there was the fact that not many particles could be analyzed therefore statistical significance of the few particles estimated may not have been a good representation of the full population. In addition, the particles visual estimates were all done in a 2-dimentional perspective.
At the time, it was an outstanding advance in qualification of samples, but as technology advanced there would be new ways to improve on this visual estimation
New Automated Methods
Since the advent of the Krumbien and Sloss Chart, there have been various new techniques developed and commercialized for particle size however it was not until the past few decades that more automated shape measurement systems have been developed. More automated methods have allowed the analysis of a more representative sample being analyzed, less bias by using a consistent and computerized measurement technique and the ability to see all three dimensions of particles.
Dynamic Image Analysis is a method used to analyze the size and shape of particles while in motion. These instruments are capable of making measurements of tens of thousands of particles in seconds ensuring a representative measurement is made of the sample. In addition, this automated method eliminates user bias and, in many cases, enables a random orientation allowing a 3-dimensional analysis of all the particles measured.
Although the existence of such automated equipment exists, there has not been much published information that enables the transfer of one existing method to a more automated one. In an effort to so do, below is an attempt to take the existing Krumbien and Sloss Chart and transfer the method to Dynamic Image Analysis.

Based on the Krumbein and Sloss Comparison chart for Roundness and Sphericity, shown below, it is difficult to understand a difference between what “roundness” is and what “sphericity”” is. Roundness seems to be a reference to a 2-dimentional image and how close it is to a round circle. Sphericity seems to be a reference starting from a flat particle, in 2 dimensions to a round particle or elliptical particle.
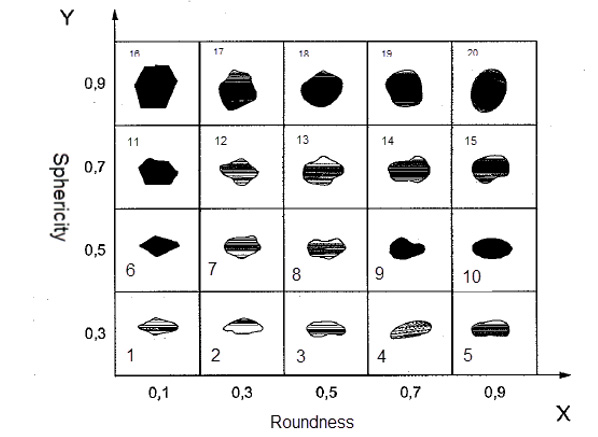
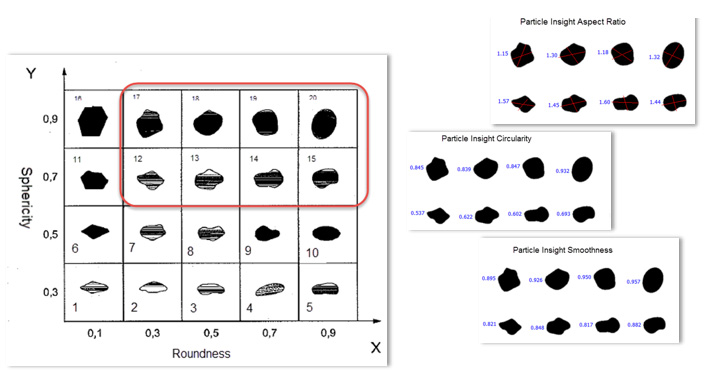
To best compare this to Dynamic Image Analysis, the individual images in the Krumbein and Sloss chart have been numbered as shown below and visual observations need to be documented that can be transferred to dynamic image analysis.
Making a visual observation, by looking at the larger particles, as “Roundness” increases, or for example image #16 to #20, the particles are getting Smoother BUT their aspect ratios remain similar. Also, as particles increase in “Sphericity” or for example as they go from image #1 to #16, the particles seem to be increasing in Circularity AND their aspect ratios seem to get closer to a 1:1 ratio.
.
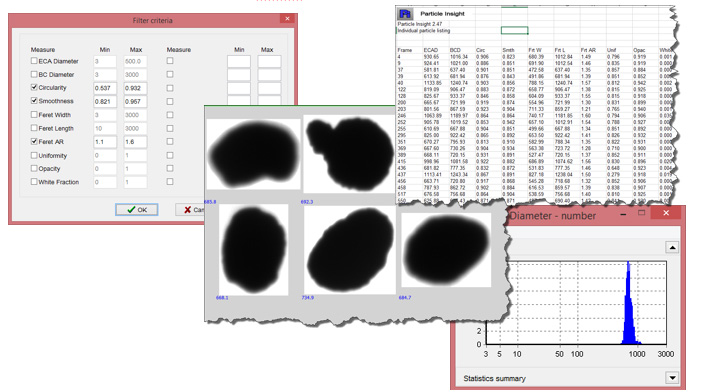
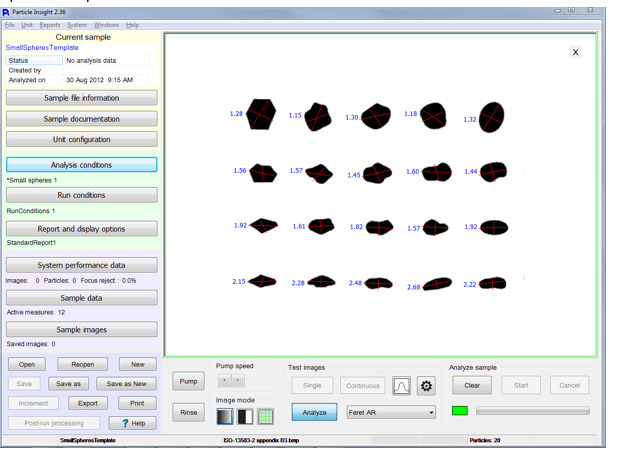
A high-resolution scan was made of the Krumbein and Sloss chart and processed using the software of a dynamic image analysis system, the Particle Insight. Below we see the scanned image and the application of the Feret Aspect Ratio shape measure.
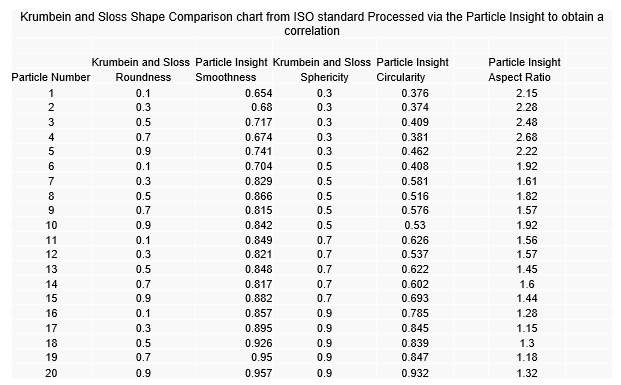
Because the visual observation of the Krumbein and Sloss chart seems to be a selection of Circularity, Smoothness and Aspect Ratio, the indivisual Krumbein and Sloss particles were all analyzed using the Particle Insight software for each measure and tabulated.
X Axis Equivalence………………
Assuming that as the “Roundness” factor increases on the X-Axis would be equivalent to an increase in Particle Insight “Circularity” shape measure is not the case. As we can see there are some particles that as they go from left to right (increasing “roundness”) have some decreasing Circularity
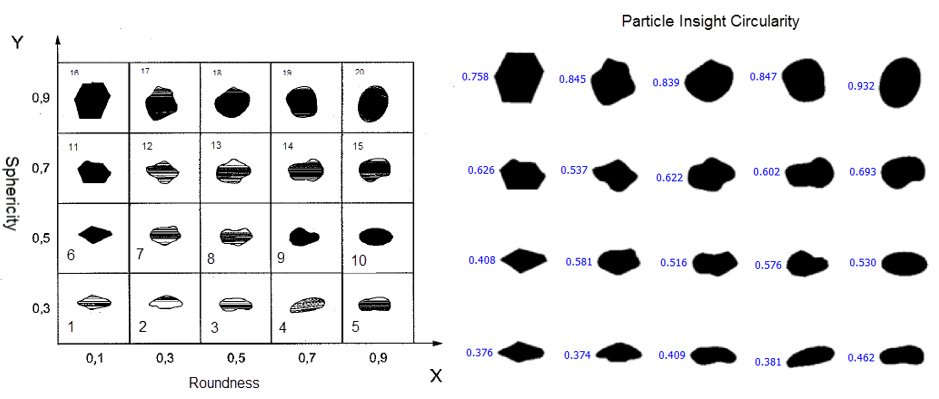
Y Axis Equivalence………………
Assuming that as “Sphericity” factor increases on the Y-Axis would be equivalent to an increase in the Particle Insight “Smoothness” shape measure is also not the case. As we can see there are some particles that as they go top to bottom, (increasing “Sphericity”) have some decreasing Smoothness values.
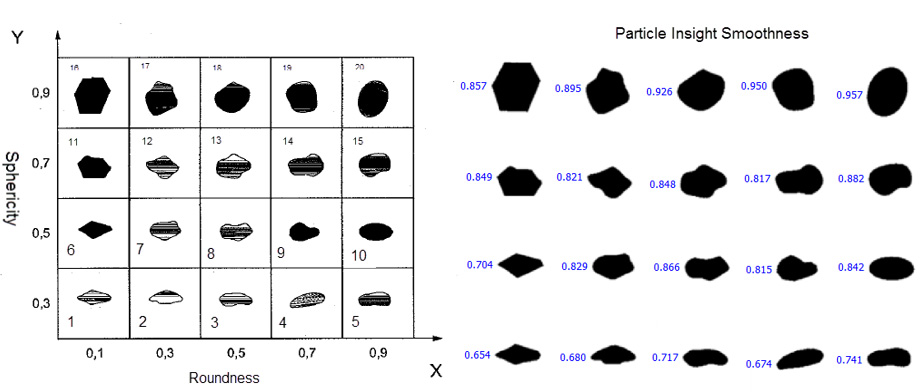
Y Axis Equivalence………………
However, it is clear to see that an equivalent measure to the Krumbein and Sloss Comparison chart for “Sphericity” (Y-axis) would be Aspect Ratio. See below.
Therefore, for the Y-Axis the Particle Insight would be able to automate and replace the Sphericity measure with Aspect Ratio and the X-Axis would be a combination of Circularity and Smoothness.
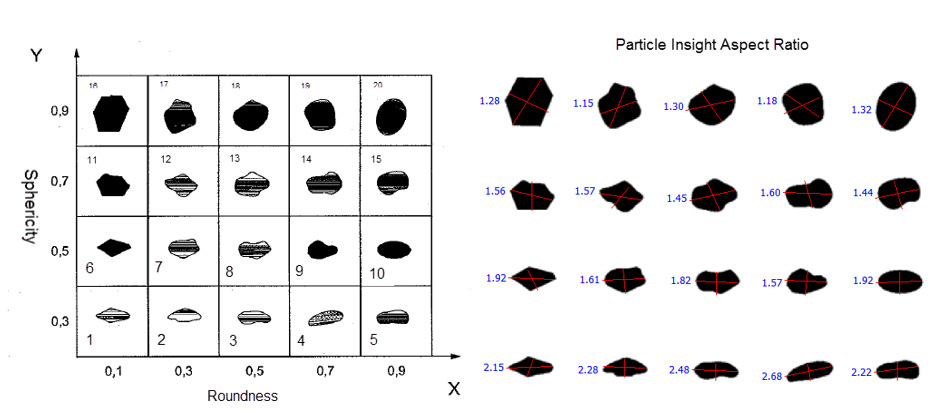
Practical Example of K&S conversion to Dynamic Image Analysis
To apply this conversion to a practical application we show here a Bauxite Frac Sand sample classified and sold to the public as a #20/40 product. This classification indicates that the particle size is expected to be between a 20 mesh sieve and a 40 mesh sieve. This is an equivalent of 850 and 425 microns in equivalent circular diameter in size.
It is important to keep in mind that classification by general sieves is done by stacking sieves and once the segregation is completed, the weight in each sieve is used to determine what percent of the population is in each size class. It is also important to realize that this method also assumes that particles are spherical in nature. However, as will be shown, not all particles are spherical.
The particles being analyzed here are described as a synthetic bauxite-based proppant with medium mechanical strength which is suitable for fracturing gas and oil wells involving contained high pressures up to 10,000 psi.

Sieve Correlation for Size
Many applications require results based in Sieving methods. Here below we report the size of a Frac Sand sample based in not only size assuming particles are around (Equivalent Circular Area Diameter) but also correlated to Sieve Analysis.
By having an automated method, such as Dynamic Image Analysis, to give more detail on many shape measurements, including size and correlation to sieve results, enables the end user to transfer older methods (Sieving) to the more automated methods.
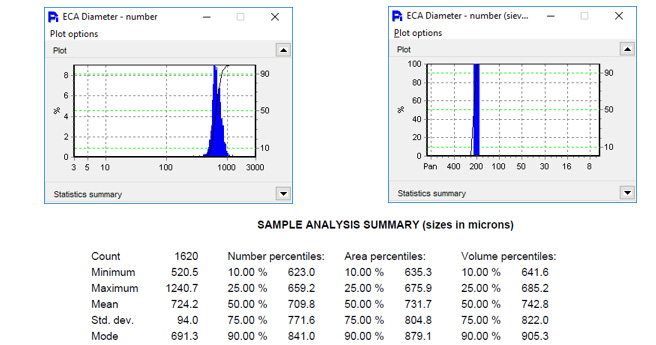
Sieve Correlation for Size
Below are a few of the thousands of particles analyzed. As can be seen the sizes shown, many are relatively round but some are not. Some also have larger aspect ratios than others.
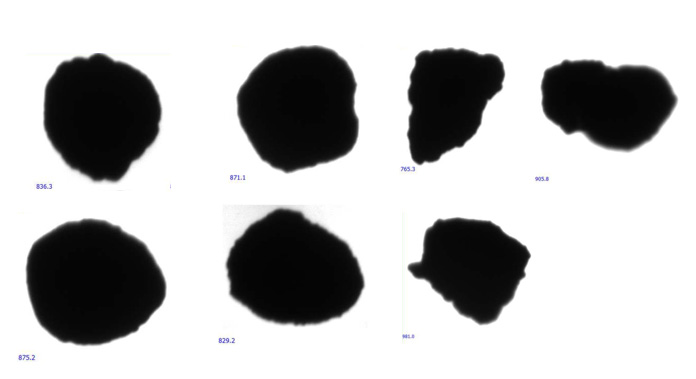
Correlation for Shape
Applying Dynamic Image Analysis to the Krumbein Sloss Chart we are able to obtain more exact measures of not only Circularity and Size but also Smoothness.
With that in mind we will select particles itemized as #12 through #20 identified in the Krumbien and Sloss Chart. Using the analyzed equivalents, we can obtain the values of these select particles using the Particle Insight software limits.
Correlation for Shape
After analyzing the Krumbien and Sloss Chart with the Particle Insight, the shape limits for the desired shapes are entered into the filter criteria to analyze the sample results based on the desired limits. From this particle listings, thumbnail images and histogram data can be analysed for each particle that meets the criteria,